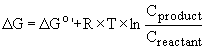
GIBB'S FREE ENERGY AND THE NATURE OF CHEMICAL REACTIONS
Introduction: In a chemical reaction, some bonds are broken in the reactants in order to form bonds in the products. Some product molecules will participate in the reverse reaction in order to reform reactants. The initial rate of product formation depends on the initial concentrations of reactants and products. As product concentration increases, the rate of the reverse reaction increases. Eventually, the rate of forward and reverse reactions become equal. Under these circumstances, the concentrations of reactants and products are constant, and the mixture is said to be in chemical equilibrium. Since breaking bonds requires energy and forming bonds releases energy, the net energy of a chemical reaction will depend on the sum of energy absorbed and generated. Eventually, the difference in energy between the reactants and products decreases as equilibrium is achieved.
Importance: We can look at the energy of a chemical reaction to predict the net direction of a chemical reaction and understand how changes in energy are related to achieving chemical equilibrium. By quantifying the free energy of reactants and products we can estimate the direction and nature of a chemical reaction.
Questions: How can we measure the energy of a chemical reaction? How can we predict the direction of a chemical reaction? How do properties of the reactants and products affect the net result of a chemical reaction?
Variables:
|
G |
Gibb's free energy (kcal/mol) |
|
D Go' |
Standard free energy (kcal/mol) |
|
H |
heat energy (kcal/mol) |
|
T |
temperature (Kelvin) |
|
S |
entropy (kcal/mol K) |
|
R |
universal gas constant (1.987 cal/mol K) |
|
cproduct, creactant |
concentration of product, reactant (M) |
|
Keq |
equilibrium constant |
Methods: A measure of free energy, the potential energy of a reaction, can be used to predict properties of chemical reactions. In the late 1800's, J. W. Gibbs showed that free energy (G) of a system can be defined as
G = H - TS
where H is the heat energy of the system, T is the temperature, and S is entropy. Heat energy (H) is a measure of the energy in a chemical bond: tightly bound molecules have higher heat energy. Entropy (S) is a measure of the disorder in a system. Molecules distributed randomly have high entropy (large S) while ordered molecules have low entropy (small S).
Every chemical reaction results in a change in free energy which we can measure as
D
G = Gproducts - Greactants = Hproducts - Hreactants - T(Sproducts - Sreactants) = DH - TDSA chemical reaction will have a DH < 0 if the heat energy of the reactants is greater than the products. A reaction will have DS < 0 if the reaction results in increased order and DS > 0 if the reaction results in increased entropy.
The net direction of a chemical reaction will be from higher to lower energy. In other words, if the energy of the reactants is higher than the energy of the products, Greactants > Gproducts, the reaction will occur spontaneously. In such a case, DG < 0, and the free energy of the system decreases with the reaction. In the opposite case, DG > 0, and energy is required for the reaction to occur.
In a mixture of reactants and products, the chemical reaction and its reverse occur until chemical equilibrium is achieved. If we begin with a large concentration of reactants, the free energy of reactants is much greater than products and the reaction proceeds. As the concentration of reactants decreases as products are formed, the difference in free energy decreases until the free energy of the products and reactants are equal. Therefore at chemical equilibrium, DG = 0.
Clearly, the free energy of a chemical reaction depends on the heat energy and entropy of the reactants and products. Free energy also depends on the concentration of reactants and products. This is because the movement of molecules from a more to less concentrated state can perform work. Reference books refer to DGo' as the standard free energy of a reaction when temperature is 298 Kelvin, pressure is 1 atm, pH is 7.0, and initial concentrations of reactants and products are equal. Some examples are given in the following table.
|
Chemical Reaction |
D Go' (kcal/mol) |
|
|
photosynthesis |
6CO2 + 6H2O --> glucose + 6O2 |
+686 |
|
hydrolysis of sucrose |
Sucrose + H2O --> glucose + fructose |
-7.0 |
|
conversion of ATP to ADP |
ATP + H2O --> ADP + phophate |
-7.3 |
|
esterification |
glucose + phosphate --> glucose 6-phosphate + H2O |
+3.3 |
When the concentrations of reactants and products are variable, we can determine DG as
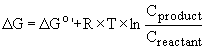
where R is the universal gas constant, T is temperature, and Cproduct, Creactant are the initial concentrations of the products and reactants.
We can plot DG as a function of Cproduct/Creactant to see how the free energy of the reaction changes as reactants are converted to product (Cproduct/Creactant increases). As an example we will look at chemical reaction of photosynthesiswhich has a standard free energy DGo' = +686 kcal/mol. The reverse reaction has DGo' = -686 kcal/mol.

Interpretation: When the concentration of the reactants is much greater than the products (Cproduct/Creactant much less than 1), for both photosynthesis and the reverse reaction, DG is negative so the reaction progresses spontaneously. For the reverse reaction, as the concentration of product increases, the reaction approaches a chemical equilibrium where DG = 0. For photosynthesis, however, as the amount of product increases, DG quickly becomes positive even though less than half of the reactants have been converted to product (Cproduct/Creactant < 0.5). In other words, for more product to be created, this reaction is not spontaneous, and energy is required for the chemical reaction to occur.
We can also use this equation for DG as a function of product and reactant concentrations to determine the equilibrium constant of a reaction. The equilibrium constant (Keq) is Cproduct/Creactant when chemical equilibrium is achieved (DG = 0). By setting DG = 0 and solving for Keq we find
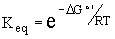
We can plot Keq as a function of DGo', we can see how the equilibrium constant is affected by the standard free energy of a chemical reaction.
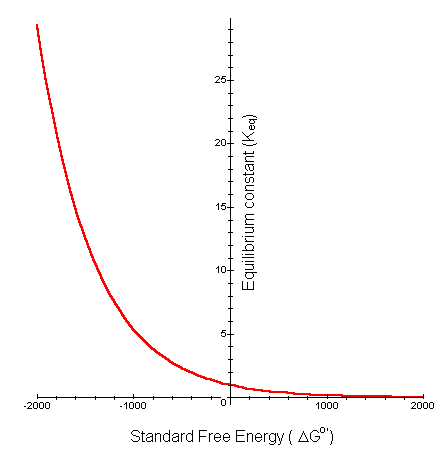
For chemical reactions where the free energy of the reactants is much greater than the free energy of the products (DGo' < 0), the reaction proceeds spontaneously and the net result is a large ratio of product to reactant (large Keq). For reactions where DGo' > 0, very little of the reactant may be converted to product without the input of additional energy into the system.
Conclusions: The rate and direction of a chemical reaction depends on the free energy, entropy, and concentration of the reactants and products as well as the temperature and pH of the system. Chemical reactions progress in the direction of high to low energy. We can estimate the direction of the chemical reaction, as well as the equilibrium concentrations of reactant and product, by examining the energy of the reactants and products.
Additional Questions:
1. What kind of function is DG as a function of DH? What would -TDS give us? What direction does the function shift if the entropy of the chemical reaction increases from reactants to products (DS increases)? What change does this result in for DG?
2. Look at the equation for the change in free energy with a chemical reaction (DG). Under what conditions for DH, T, and DS would you expect a spontaneous reaction (DG < 0)? For example, if DH > 0, is it still possible for the reaction to be spontaneous?
3. Verify that DG = DGo' when the initial concentrations of reactants and products are equal.
4. Verify the equation for Keq as a function of DGo'.
5. Look at the table of standard free energies for different chemical reactions. Assuming T = 298 Kelvin, calculate the equilibrium constant (Keq) for each reaction. Which of these reactions would occur spontaneously?
Sources: Darnell, J., H. Lodish, and D. Baltimore. 1986. Molecular Cell Biology. Scientific American Books, Inc., New York
Tobin, A. J. and R. E. Morel. 1997. Asking About Cells. Harcourt Brace & Company, New York.
Copyright 1999 M. Beals, L. Gross, S. Harrell