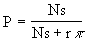
CHEMORECEPTION
Introduction: Chemoreception occurs when specific receptors in the cell membrane bind with diffusing chemicals in the surrounding medium. For example, bacteria such as E. coli and Salmonella often navigate in response to chemical attractants or repellants.
Importance: A cell that depends on chemoreception must devote a certain amount of its resources and surface area to building receptors. Building a large number of receptors throughout the cell membrane certainly increases the cell's ability to detect a chemical, but it is also costly to the cell. We can use simple equations and graphs to examine how a cell can have adequate chemoreception without high cost.
Question: Should a cell invest a large amount of resources and surface area in building chemoreceptors in its cell membrane in order to detect chemicals?
Variables:
|
P |
probability of a molecule finding a receptor |
|
N |
number of receptors in cell membrane |
|
s |
radius of receptor (micrometers) |
|
r |
radius of spherical cell (micrometers) |
Methods: For a spherical cell of radius r, the probability (P) that a molecule that has collided with the cell will find a receptor can be expressed as
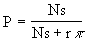
where N is the number of receptors in the cell membrane and s is the radius of each binding site. As the number of sites (N) gets very large, 100% of the molecules are likely to collide with a receptor.
We will compare the probability of chemoreception with the amount of cell surface area devoted to receptors. The surface area of a spherical cell is given by 4pr2 and the area of a binding receptor is given by ps2. The proportion of the cell's surface area covered by receptors can be estimated as N(ps2)/4pr2.
By graphing these two equations we can gain a lot of information. Assume a receptor radius of 10 angstroms (1 micrometer = 108 angstroms) and a cell radius of 10 micrometers.
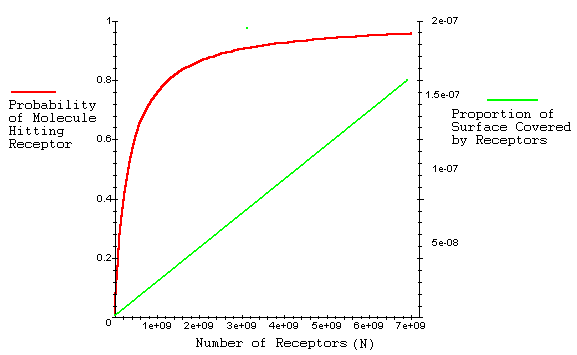
The probability of a molecule colliding with a receptor, graphed as a function of N, increases rapidly at first and then asymptotically approaches a probability of 1.0 as N gets very large. The proportion of the cell's surface area covered by receptors increases linearly.
Interpretation: Certainly for very large N, the cell is well covered by chemoreceptors, and almost 100% of diffusing molecules are likely to bind. However, a small number of receptors, taking up considerably less area, have a probability almost as high due to the quickly increasing nature of this function. Because of the asymptotic nature, above a certain number of receptors, the probability of binding only increases slightly by having additional receptors. Yet the cell gain s the additional burdens of building chemoreceptors and losing surface area.
Conclusion: One expects a cell to have optimum performance (a high probability of chemodetection without a high cost) when only a small fraction of the cell surface has chemoreceptors. A cell can devote only a minor amount of resources to building receptors, but still be able to detect chemicals in its surroundings.
Additional Questions:
1. Look at the probability (P) equation again. Formally calculate the horizontal asymptote. What happens as N goes to infinity?
2. How would our analysis change if a cell could only have a limited number of receptors (N is fixed), but could vary their size (s)?
Source: Berg, H. C. and E. M. Purcell. 1977. Physics of chemoreception. Biophysical Journal 20:193-219
Copyright 1999 M. Beals, L. Gross, S. Harrell